Measurements of thermal resistance of a tube NF EN ISO 8497 December 1996 Thermal insulation – Determination of properties relating to steady-state heat transfer in thermal insulation for pipes
Posté le 2 March 2022 dans News
We recently carried out standardized measurements to characterize the performance of an insulation system for pipes carrying a fluid. We carried out these measurements according to standard NF EN ISO 8497.
This standard specifies a method for measuring thermal properties relating to steady state heat transfer through pipe insulation at temperatures above ambient. It standardizes the method of measurement, including the operating modes and the operation of the apparatus.
This is typically the case for district heating networks and CTA systems or air-air heat pumps.
The method :
The principle is to heat the inside of the test tube and to measure the temperature differences between the inner skin and the outer skin of the insulation system. In our work we extracted the average thermal conductivity of the insulation.
To simulate the transport of air inside and ensure the highest possible and constant convection coefficient over the entire length of the test tube, we have created circulation using a ventilation system:
Plan of the device:
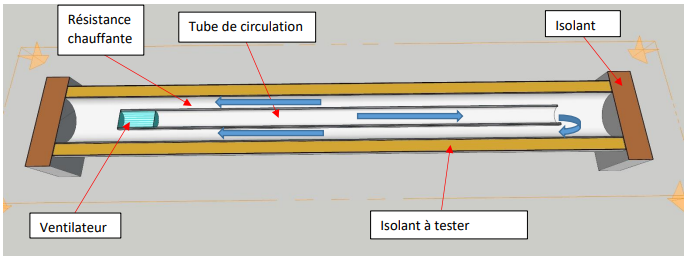
In the diagram the blue arrows represent the convection air flow. Centering guides keep the circulation tube central.
A fan is placed inside the circulation tube. Thermocouples are fixed on each tube in order to check the homogeneity of the temperature in the central tube.
An external ventilation system makes it possible to have a convection coefficient independent of the temperature of the tubes.
Placement of the different thermocouples:
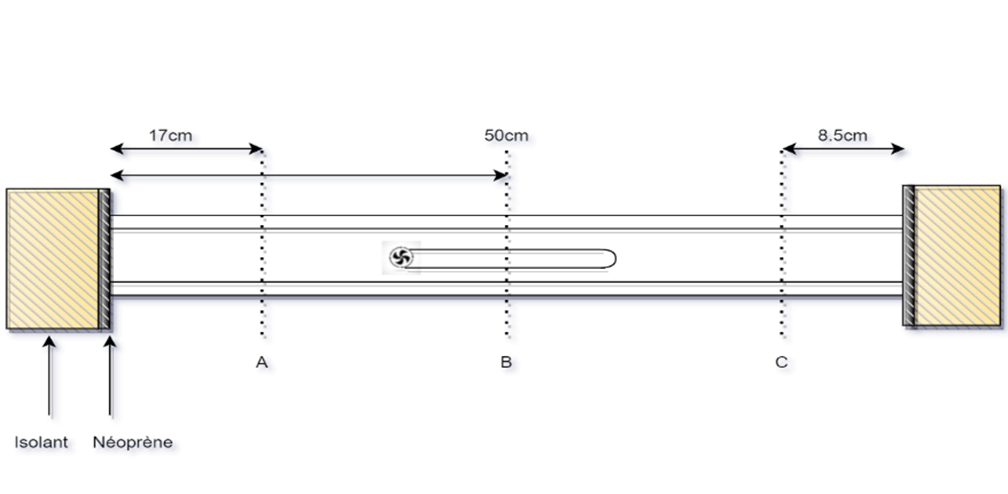
Calculation of average thermal conductivity:
The effective power takes into account the heating power and the fan power. Heat losses are calculated according
to the standard.
Losses are estimated by calculation:
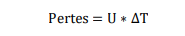
With U the loss coefficient estimated according to the guard insulations:
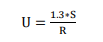
With R the thermal resistance of the insulation of the guardR = 2.6 (K. m²)/W
And S the surface of the inner tubeS =
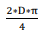
Linear resistance on a 1m tube [K/W]:
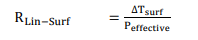
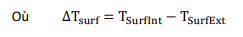
With TSurfExt : Average of the 12 surface temperatures outside the outer tube
And TSurfInt : Average of the 12 internal surface temperatures of the inner tube
Average thermal conductivity [W/ ( mK )]:
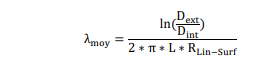
Or
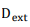 is the outer tube diameter (m)
is the outer tube diameter (m)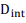 is the inner tube diameter (m)
is the inner tube diameter (m)- L is the length of the tube (m)
Surface thermal resistance [K. m2/W]
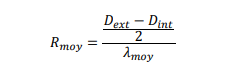
Or :
- 𝐷𝑒𝑥𝑡 is the outer tube diameter (m)
- 𝐷𝑖𝑛𝑡 is the inner tube diameter (m)
- 𝜆𝑚𝑜𝑦 average thermal conductivity [W/ ( mK )]
